Category: Elementary math

Thinking in the Concrete~Manipulatives in Math Class

Bridget Dunbar (@BridgetDunbar)1 Comment

Amie Albrecht retweeted this the other day over a year ago (I just came back to finish this post):

It reminded of me when I used my son to try out the first part of this NRich problem:

Screen Shot 2016-06-26 at 8.19.28 PM

I’ve used this problem with middle school students before…but I wanted to use it in a math for elementary teachers undergraduate class, so I needed to see how an elementary student would think it through (he’s in 5th grade).

He attempted some mental thinking about the problem at first. But, I could tell he was going to get frustrated. I found something that could work as counters for him and asked him to represent the problem. He argued with me about it and finally said “only babies use these.”

I pushed him to use the counters…and he figured the problem out quite simply.

Using manipulatives makes problems so interesting to think about. But, I just asked him about that day and he agreed that the tool made the problem easier, but he didn’t like using them.

We need to use more manipulatives in math class!

Elementary Observations from a Secondary Math Teacher

Bridget Dunbar (@BridgetDunbar)2 Comments

Here is the interaction that I had with my 5th grade son yesterday:

Me: “What does 6 ÷ 2 mean?”

Son: “Six split into two equal groups.”

Me: “What else could 6 ÷ 2 mean?”

Son: [crickets]

Me: “…how many groups of two are in six… so…what does 6 ÷ ½ mean?”

Son: “…how many groups of one-half are in six…12.”

Me: “What is 6 ÷ ¼?”

Son: “…how many groups of one-fourth are in six…24.  I remember this every time you remind me…but I always forget.”

My son is very strong with fractions, and he’ll go straight to invert and multiply if I let him.  I often engage him in this same conversation…working on the understanding of dividing a whole number by a unit fraction because I know how difficult division of fractions can be for students.

My hypothesis is that it has to do with the two interpretations of division:

(a) How many groups?                      and                     (b) How many in each group?

***At this point…I will hope that elementary people will tell me I’m wrong if I’m wrong…

I began teaching Math for Teachers at the local college (St. Mary’s College of Maryland~my alma mater) two years ago.  I use Sybilla Beckmann’s Math for Elementary Teachers fourth edition for this course.

The first activity for the division unit is this:

Write a simple word problem and make a math drawing that you could use to help children understand what 10 ÷ 2 means.

Each year I’ve taught this course EVERY single student wrote a how many in each group problem.  This is called the sharing model of division.

I think it’s less natural for students to consider the how many groups (or measurement model) interpretation of division.  But it seems that this is the model that makes the most sense for the division of fractions.

I think this less familiar interpretation of division can also impact a student’s ability to be successful with long division.

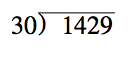
Screen Shot 2016-06-05 at 1.01.19 PM

A student needs to be able to think, “How many groups of 30 are in 1429??”

I think it’s important to be purposeful with the language we’re using with students, and how we are exposing them to different interpretations of division specifically.  Secondary math teachers can learn A LOT by digging into the elementary material.